Relevant Concepts
Two important concepts are repeatedly used in photogrammetry: a) coordinate transformation b) the collinearity condition. Coordinate transformation helps in relative and inner orientation (i.e. mainly for 2D transformation) and the collinearity condition plays a part in 3D stereo model formation and in orthorectification.
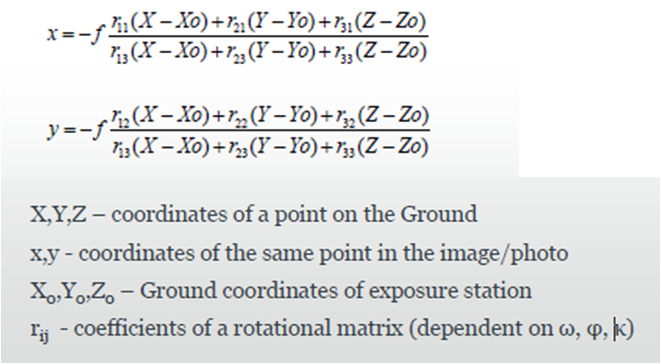
The Concept of Collinearity
The condition of collinearity is satisfied when the exposure station of the photograph, the object point on the ground and its corresponding point in image are all in a straight line. Based on this concept, several complex mathematical relationships can be derived. The mathematical expression of the collinearity condition is given below as equations:
In this equation there are 9 unknown parameters, i.e. X, Y, Z, ω, φ, κ, X0, Y0 and Z0; and three known parameters, i.e. x, y (i.e. the coordinates of a point on the photo), and f (focal length of the camera, which is approximately 152mm). A single ground control point (GCP) will feed into the above equations (for X,Y,Z) and we will get 2 equations per point, and hence in order to solve the 6 remaining unknowns, assuming the absence of GPS which would measures position and an Inertial Measurement Unit (IMU) which would measure camera rotation angle, X0, Y0 and Z0 ; ω, φ, κ are not known, we need only 3 GCPs, and the 4th GCP will help achieve fine refinement.
The Concept of Space Resection
This is the process of identifying exposure station location on the ground (i.e., X0, Y0 and Z0). If we knew these ground coordinates it would be easy to solve the colllinearity equation. In the absence of ground coordinates (i.e. when there is no ground reference) we have to find an alternative approach. In such cases we need two aerial photos, and one of the photos can be considered as having 0 values for all its camera coordinates (i.e, for ω, φ, κ, X0, Y0 and a constant Z0). The process of determining the point coordinates of the object lying in the stereo overlap area of the two photos of a stereo pair is designated as the process of space intersection. The basic principle behind this concept is that the corresponding rays to a common object point from two stereo photographs must intersect at a common point. This determination requires the parallax equation previously discussed. The following figure depicts the process of space intersection:
References
Baltsavias, E., Zhang, L., Holland, D., Srivastava, P. K., Krishna, B. G. & Srinivasan, T. P. (2006) Extraction of geospatial information from high spatial resolution optical satellite sensors http://www.isprs.org/education/PDF/GOA_BGK_Orthoimage_Section5.pdf